- Home
- Math Notes
- Calculus I
- Common Functions
Hyperbolic Functions
Hyperbolic cosine is y=cosh(x)=2ex+e−x.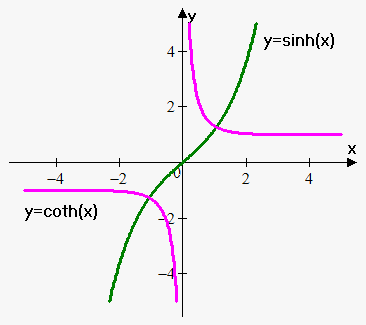
Hyperbolic sine is y=sinh(x)=2ex−e−x.
Hyperbolic tangent is y=tanh(x)=cosh(x)sinh(x)=ex+e−xex−e−x.
Hyperbolic cotangent is y=coth(x)=sinh(x)cosh(x)=ex−e−xex+e−x.
Hyperbolic secant is y=sech(x)=cosh(x)1=ex+e−x2 .
Hyperbolic cosecant is y=csch(x)=sinh(x)1=ex−e−x2.
There is some similarity between hyperbolic functions and trigonometric.
 Domain of hyperbolic functions is (−∞,∞), except for function y=coth(x) which is undefined when x=0.
Domain of hyperbolic functions is (−∞,∞), except for function y=coth(x) which is undefined when x=0.
Formulas that hold for any x and y:
- cosh(x±y)=cosh(x)cosh(y)±sinh(x)sinh(y).
- sinh(x±y)=sinh(x)cosh(y)±cosh(x)sinh(y).
- cosh2(x)−sinh2(x)=1.
- cosh(2x)=cosh2(x)+sinh2(x).
- sinh(2x)=2sinh(x)cosh(x).
This formulas can be easily proved using definitions of hyperbolic functions.

 Domain of hyperbolic functions is , except for function which is undefined when .
Domain of hyperbolic functions is , except for function which is undefined when .