1. Home
  2. Math Notes
  3. Calculus I
  4. Common Functions

Rational Function

Function of the form f(x)=Q(x)P(x)=a0xn+a1xn−1+…+an−1x+anb0xm+b1xm−1+…+bm−1x+bm{f{{\left({x}\right)}}}=\frac{{{Q}{\left({x}\right)}}}{{{P}{\left({x}\right)}}}=\frac{{{a}_{{0}}{{x}}^{{n}}+{a}_{{1}}{{x}}^{{{n}-{1}}}+\ldots+{a}_{{{n}-{1}}}{x}+{a}_{{n}}}}{{{b}_{{0}}{{x}}^{{m}}+{b}_{{1}}{{x}}^{{{m}-{1}}}+\ldots+{b}_{{{m}-{1}}}{x}+{b}_{{m}}}}, where Q(x){Q}{\left({x}\right)} and P(x){P}{\left({x}\right)} are polynomials is called rational function.

Domain of this function consists of all x{x} such that Q(x)≠0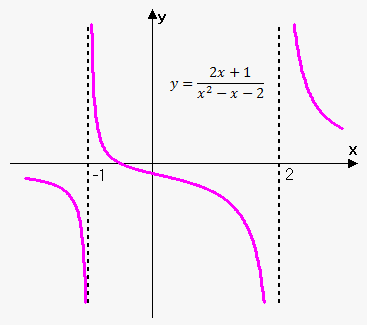{Q}{\left({x}\right)}\ne{0}.rational function

Simple example of the rational function is f(x)=2x+1x2−x−2=2x+1(x−2)(x+1){f{{\left({x}\right)}}}=\frac{{{2}{x}+{1}}}{{{{x}}^{{2}}-{x}-{2}}}=\frac{{{2}{x}+{1}}}{{{\left({x}-{2}\right)}{\left({x}+{1}\right)}}}. Its domain is all x{x} except x=2{x}={2} and x=−1{x}=-{1}.

This function is shown on the figure.